|
|
The importance of the problem of rectilinear motion in
those days is witnessed by the over 150 articles that
appeared during the nineteenth century on this topic, in journals of
mechanics and mathematics. A great
mathematician, the Russian Tchebycheff, who is credited
with fundamental results in probability theory and in
number theory, dedicated much energy to finding an
"exact" solution to the problem. In 1850,
Tchebycheff managed to determine another approximated
solution to the problem which may have been more accurate than Watt's,
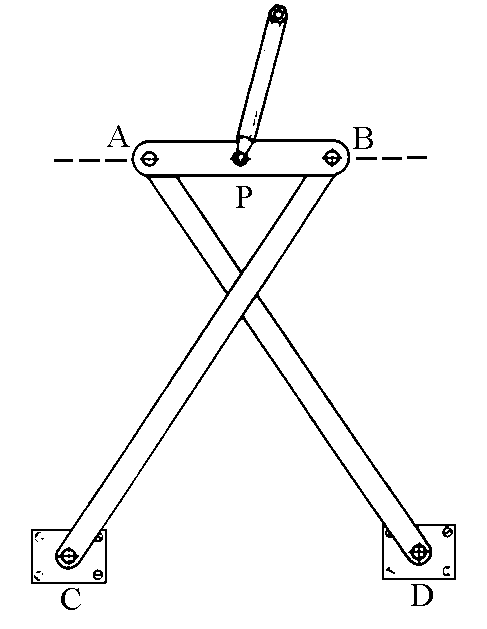
but was certainly less practical. It is based on a system
with 3 connecting rods DA, AB, BC with C and D fixed, BC
= AD and with distances such that AD : CD : AB = 5 : 4 :
2 . Around the symmetrical position shown in Figure 4,
the median point P of AB draws a substantially
rectilinear line (although the complete trajectory of P
looks like a semicircle!). Sylvester writes that, after
many fruitless efforts, Tchebycheff concluded that
there existed no mechanism which could give an exact
solution to the problem.
Tchebycheff's mechanism was used to drive the motion of
the blades of timber saws.
It was a pupil of Tchebycheff, Lipkin, who found the
mechanism that solves the problem in an exact manner, but
he was preceded by Peaucellier, a French army officer
whom we will discuss shortly.
|

|
n the meanwhile, the Englishman Roberts
had proposed another approximate solution, based on a
three-rod mechanism and on a BPC blade in the shape of an
isosceles triangle. In this case one must have AB =
BP = PC = CD and AD = 2
BC , the vertex P of the blade traces for a
remarkably long tract a nearly straight line.
|