In fact, burning mirrors and the mirage are made not with parabolas, but with surfaces obtained rotating parabolas around their axis. These surfaces are called rotation paraboloids. A paraboloid can be obtained rotating a liquid fast enough inside a cylindrical container. If instead the liquid is contained between two close planes, we have a parabola.

Similarly, when we rotate an ellipse or a hyperbole, we obtain a rotation ellissoid or hyperboloid.

These surfaces also have reflecting properties similar to the ones of the paraboloid. We have built an elliptical chamber, obtained by rotating a half-ellipse along the axis. A phenomenon similar to the burning mirror happens in it: if we place ourselves in one of the foci and we speak towards the elliptical wall, even in a very low voice, those who are in the other focus receives the voice very clearly, while whoever is in between hears hardly anything.

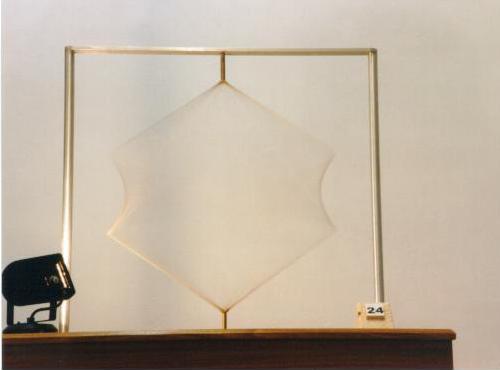
The rotation hyperboloid has the notable characteristic of being a lined surface, that is of being constituted only of straight lines, as we can see from the hyperboloid obtained with strings.

This produces an unexpected phenomenon: rotating a straight line opportunely inclined, one can make it go through a hyperbole-shaped slit. The rod, rotating, describes a hyperboloid, which, intersected with a plane, leaves us with the two slits through which the rod passes with no difficulty.

The same surface is obtained by rotating a cube. While the upper and lower edges, that meet the rotation axis, form a cone, the intermediate ones, which do not meet the axis, generate a hyperboloid, which is visible thanks to the persistence of images on the retina.