|
>Reflected or refracted rays are all
tangent to the caustic they form. More in general, if one
takes a family of straight lines (as in the caustics the
reflected or refracted rays), they can be uniformly
distributed on the plane, as it happens when for example
they are parallel, but they can also concentrate onto a
curve, to which they are all tangent. This curve is
called the envelope of the lines. Thus a reflection
caustic is the envelope of the rays reflected by a
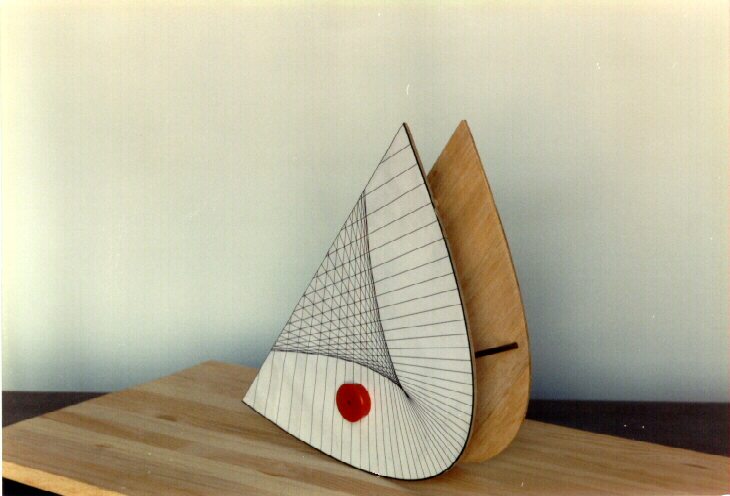
mirror. One can create envelopes of lines by pulling
strings between various points on the plane or in space.
The rotating tetrahedron at the entrance, the bicycle
wheel above your head, the perpendicular lines to the
parabola, show curves obtained as envelopes of straight
lines.
To build an envelope one need not start with
straight lines - the same can be done with curves. Every
curve of the family will be tangent to the envelope, that
is it will have the same tangent line in the contact
point. On the computer, it is possible to see various
examples of envelopes of circles and also of more complex
curves.
An interesting example comes from ballistics. Ignoring
the resistance of air, the shell shot by an artillery
piece will describe a parabola, the shape of which will
depend on the power of the cannon and on the shooting
angle. Let us suppose now that we have a cannon which
always shoots with the same power, but that can vary the
angle. Where must one stand to be sure one is outside the
cannon's range?
The answer is simple: one traces all the shells'
trajectories with varying angles, and one stands outside
the region these cover. This region is delimited by the
curve enveloping all the parabolas, which in this case is
also a parabola.
Let us note that the caustics, and envelopes in
general, are not curves that physically exist. What exist
are the rays of light, or more in general the lines of
the family. The curve that they envelop appears only
because they concentrate on it. Thus the light rays
concentrating on the caustic shed more light on the area
of the plane corresponding to the curve, and they draw
it. The same happens with the lines normal to the
parabola, which can be seen in the entrance hall. They
trace a curve that can be seen, although it does not
exist - only the lines enveloping it exist.
In some cases it is important to avoid
the formation of caustics, and to have a light which is
as uniform as possible. For example, in photography the
uniformity of illumination is essential. The Fortuny
lamp, which is still in use, thanks to the shape of its
reflecting surface, supplies a light density which is
constant in every point.
|
